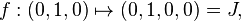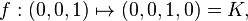Grafika:Tesseract2.png

	This Math image should be recreated using vector graphics as an SVG file. This has several advantages; see Commons:Images for cleanup for more information. If an SVG form of this image is already available, please upload it. After uploading an SVG, replace this template with template {{SupersededSVG|new image name.svg}} in this image.
Català | Česky | Dansk | Deutsch | English | Esperanto | Español | Français | Italiano | 日本語 | 한국어 | Nederlands | Polski | Português | Русский | العربية | 正體中文 | +/-

[edit] Summary

Image of a three-dimensional net of a tesseract, created by Dmn with Paint Shop Pro.

The net of a tesseract is the unfolding of a tesseract into 3-D space. Let the dimension from left to right be labeled x, the dimension from bottom to top be labeled z, and the dimension from front to back be labeled y. Let coordinates by (x, y, z). Let the top cube have coordinates (0,0,1), the cube below it have coordinates (0,0,0), the cube in front of it have coordinates (0,−1,0), the cube behind it have coordinates (0,1,0), the cube to the left (−1,0,0), the one to the right (1,0,0). Let the cube below the central one have coordinates (0,0,−1) and the one at the bottom (0,0,−2).

The central cube (0,0,0) is seen to be connected to six other cubes, but when folded in 4-D every cube connects to six other cubes. The frontal cube (0,−1,0) connects in the −Y direction to (0,0,−2), in the +Y direction to (0,0,0), in the +X direction to (1,0,0), in the −X direction to (−1,0,0), in the +Z direction to (0,0,1), in the −Z direction to (0,0,−1).

There are twelve different ways in which the tesseract can be rotated (in 4-D) by 90 degrees in such a way that four of the cubes exchange positions cyclically while the remaining four cubes stay in place but rotate (in 3-D). For example, one 4-D rotation causes the following four-cube exchange: (0,0,1)→(0,0,0)→(0,0,−1)→(0,0,−2)→(0,0,1). Meanwhile, the same rotation causes cube (0,1,0) to rotate 90 degrees around the +X axis, the (0,−1,0) cube to rotate 90 degrees around the −X axis, the (1,0,0) cube to rotate 90 degrees in the −Y direction and the (−1,0,0) cube to rotate 90 degrees in the +Y direction.

The twelve 4-D rotations are:
1: (0,0,1)→(0,0,0)→(0,0,−1)→(0,0,−2)→(0,0,1),
2: (0,0,1)←(0,0,0)←(0,0,−1)←(0,0,−2)←(0,0,1),
3: (1,0,0)→(0,1,0)→(−1,0,0)→(0,−1,0)→(1,0,0),
4: (1,0,0)←(0,1,0)←(−1,0,0)←(0,−1,0)←(1,0,0),
5: (−1,0,0)→(0,0,0)→(1,0,0)→(0,0,−2)→(−1,0,0),
6: (−1,0,0)←(0,0,0)←(1,0,0)←(0,0,−2)←(−1,0,0),
7: (0,−1,0)→(0,0,0)→(0,1,0)→(0,0,−2)→(0,−1,0),
8: (0,−1,0)←(0,0,0)←(0,1,0)←(0,0,−2)←(0,−1,0),
9: (0,0,1)→(1,0,0)→(0,0,−1)→(−1,0,0)→(0,0,1),
10: (0,0,1)←(1,0,0)←(0,0,−1)←(−1,0,0)←(0,0,1),
11: (0,0,1)→(0,1,0)→(0,0,−1)→(0,−1,0)→(0,0,1),
12: (0,0,1)←(0,1,0)←(0,0,−1)←(0,−1,0)←(0,0,1).

Each 4-D rotation has a "dual" which is perpendicular to the 3-D rotation of the stationary cubes. There are six pairs of dual (4-D) rotations:

• 1 ↔ 4,
  
• 2 ↔ 3,
  
• 5 ↔ 12,
  
• 6 ↔ 11,
  
• 7 ↔ 9,
  
• 8 ↔ 10.
  

The dual of a 4-D rotation implies, by means of the right-hand rule, how the stationary cubes are supposed to rotate in 3-D.

Since there are eight cubes and each cube connects to six other cubes, then each cube has a pair of cubes to which it does not connect: (1) itself, and (2) its opposite. Thus there are four pairs of opposite cubes:
1: (0,0,1) ↔ (0,0,−1),
2: (0,0,0) ↔ (0,0,−2),
3: (−1,0,0) ↔ (1,0,0),
4: (0,−1,0) ↔ (0,1,0).

Each pair of opposite cubes aligns itself along opposite sides of one of four orthogonal axis of 4-D space. Therefore it is possible to establish a one-to-one onto mapping f between the unfolded positions of the cubes in 3-D and the canonical coordinates of their folded positions in 4-D, viz.

The canonical 4-D coordinates have been given labels corresponding to basis quaternions (and their negatives). Using these labels, the 4-D rotations can be expressed more simply as
1: K → 1 → −K → −1 → K,
2: K → −1 → −K → L → K,
3: I → J → −I → −J → I,
4: I → −J → −I → J → I,
5: −I → 1 → I → −1 → −I,
6: −I → −1 → I → 1 → −I,
7: −J → 1 → J → −1 → −J,
8: −J → −1 → J → 1 → −J,
9: K → I → −K → −I → K,
10: K → −I → −K → I → K,
11: K → J → −K → −J → K,
12: K → −J → −K → J → K.

All of these rotations follow a pattern A→B→−A→−B→A, so that each one can be abbreviated as an ordered pair (A,B). Then, each rotation can be abbreviated furthest as the product of the ordered pair of quaternions, which yields an imaginary quaternion:
1: (K,1) = K
2: (K,−1) = −K
3: (I,J) = K
4: (I,−J) = −K
5: (−I,1) = −I
6: (−I,−1) = I
7: (−J,1) = −J
8: (−J,−1) = J
9: (K,I) = J
10: (K,−I) = −J
11: (K,J) = −I
12: (K,−J) = I

The pairs of dual quaternions are then seen to have the following properties: the products of their single-quaternion abbreviations are always unity:

• 1 ↔ 4 : K (− K) = 1,
  
• 2 ↔ 3 : (−K) K = 1,
  
• 5 ↔ 12 : (− I) I = 1,
  
• 6 ↔ 11 : I (−I) = 1,
  
• 7 ↔ 9 : (−J) J = 1,
  
• 8 ↔ 10 : J (−J) = 1.
  

Each of the twelve rotations has a pair of candidate duals, but one of them is the reversal of the rotation, i.e. given rotation (A,B), its reverse is (A, −B), so it is disqualified as the dual of (A,B), leaving only one possible dual.

[edit] Licensing

This image is a faithful reproduction of a two-dimensional work of art and thus not copyrightable in itself in the U.S. as per Bridgeman Art Library v. Corel Corp.; the same is also true in many other countries, including Germany. The original two-dimensional work shown in this image is free content because: This image (or other media file) is in the public domain because its copyright has expired. This applies to the United States, Canada, the European Union and those countries with a copyright term of life of the author plus 70 years. العربية ǀ Български ǀ Català ǀ Česky ǀ Dansk ǀ Deutsch ǀ English ǀ Ελληνικά ǀ Esperanto ǀ Español ǀ Euskara ǀ فارسی ǀ Français ǀ Galego ǀ עברית ǀ हिन्दी ǀ Bahasa Indonesia ǀ Italiano ǀ 日本語 ǀ 한국어 ǀ Kurdî / كوردي ǀ Nederlands ǀ Norsk (nynorsk) ǀ Bahasa Melayu ǀ Polski ǀ Português ǀ Русский ǀ Slovenščina ǀ Shqip ǀ Suomi ǀ Svenska ǀ Türkçe ǀ 简体中文 ǀ 正體中文 ǀ +/- Therefore, this reproduction is also subject to the same terms as the original work. العربية ǀ Česky ǀ Deutsch ǀ English ǀ Ελληνικά ǀ Español ǀ فارسی ǀ Français ǀ עברית ǀ Indonesian ǀ Italiano ǀ 日本語 ǀ 한국어 ǀ Magyar ǀ Nederlands ǀ Polski ǀ Português ǀ Românǎ ǀ Русский ǀ Slovenščina ǀ Српски ǀ Sunda ǀ Türkçe ǀ Українська ǀ 简体中文 ǀ 正體中文 ǀ +/-